|
Welcome to SudokuOne.Com Goto ==> SudokuOne Homepage |
|
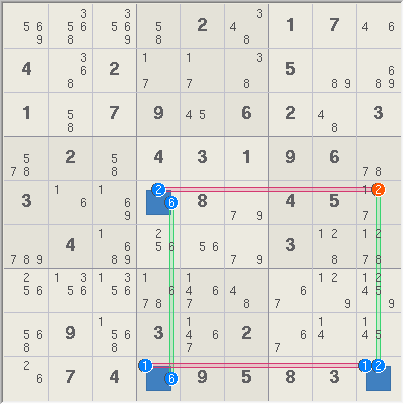
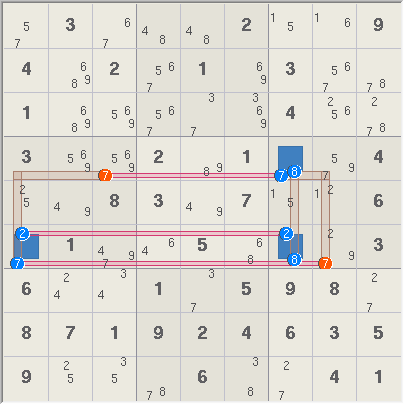
XY-Wing Points. An XY-Wing is a short chain made of three bi-value cell truths. The cell in the center is called the pivot and the other 2 cells are called pincers. An XY-Wing uses only 3 digits usually named X, Y and Z. The pivot contains the XY candidates while the pincers contain candidates XZ and YZ. Any cell that can see both pincers cannot contain another candidate for digit Z. What Sudopedia says.
|
||||||||
|
|